Projects
The LSD Collaboration uses lattice gauge theory, a proven non-perturbative numerical technique based on large-scale computation, to study strongly coupled quantum field theories in regimes which are not accessible to traditional analytic calculations. We have several active project directions related to the study of novel strongly coupled gauge theories, with applications to various models of new physics beyond the Standard Model.
Near-conformal gauge theories with many flavors
The physics of quantum chromodynamics (QCD) is known to be highly sensitive to the number of light fermion species (‘flavors’) which are active at a given energy scale. The polarization of the quantum vacuum by light flavors contributes significantly to the evolution of the measured strong coupling $\alpha_s(q)$ with the scale $q$ at which it is measured, with a ‘screening’ effect that slows its evolution.
In the Standard Model, the number of light flavors $N_f$ is equal to 3, but we can consider generalized theories where $N_f$ is larger. At a critical value $N_f^c$, the theory is expected to undergo a phase transition as the screening effect of the fermions halts the evolution of $\alpha_s(q)$ altogether, with the theory entering an ‘infrared-conformal’ phase. A significant portion of our research effort has gone into studying the nature and location of this phase transition, as well as investigating its implications for the dynamics of QCD-like theories as $N_f$ approaches $N_f^c$.
Our recent lattice calculations with $N_f = 8$ has revealed interesting dynamical results, including the presence of a light scalar $J^{PC} = 0^{++}$ bound state degenerate with the ‘pions’ of this theory, as shown below. Further study is needed to determine the explanation for the lightness of this state, and whether it may be related to the nearby transition to the infrared-conformal phase.
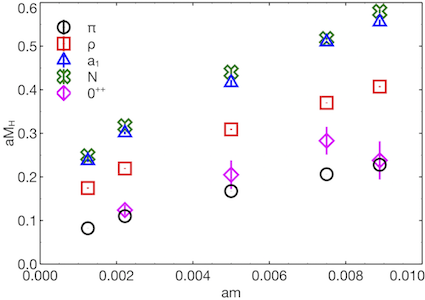
From arXiv:1601.04027, the low-lying spectrum of bound states in an SU(3) gauge theory with $N_f = 8$ light flavors. The degeneracy of the 0++ scalar state with the light $\pi$ pseudo-Goldstone bosons is in sharp contrast to QCD, and raises intriguing questions about the underlying effective field theory at low energies.
Composite dark matter
Based on a wide variety of experimental observations in astrophysics, there is strong support for the existence of particle dark matter as an important and pervasive component of our Universe. A major effort in the high-energy physics community is underway to hypothesize models of new physics which provide a dark matter candidate, and then to carry out experimental searches for their signatures.
An intriguing class of models are ‘strongly coupled composite dark matter’, in which a hidden non-Abelian gauge force gives rise to a dark matter candidate as a bound state; its stability can be naturally guaranteed by an accidental symmetry of the theory, analogous to the stability of the proton in the Standard Model. Although the dark matter itself must be overall neutral with respect to the Standard Model based on current experimental evidence, like the neutron its constituents may carry Standard Model charges, allowing for interactions with the visible sector in the early Universe in order to yield the correct relic dark matter abundance.
We have recently carried out detailed studies of a template composite dark matter model, dubbed ‘stealth dark matter’. This model is based on an SU(4) gauge theory, with four species of electroweak-charged fermions. The model possesses a high degree of symmetry, resulting in the suppression of leading interaction of the baryon-like dark matter candidate with the Standard Model force carriers. For example, the dark matter’s leading interaction with the photon in this model is through its electromagnetic polarizability, a two-photon interaction. The polarizability of the dark matter candidate in this strongly coupled theory can be computed from first principles on the lattice, leading to experimental bounds as depicted in the plot below.

From arXiv:1503.04205, bounds on the detection of ‘stealth dark matter’. The purple band shows the predicted direct-detection cross section for stealth DM through its electromagnetic polarizability, which was calculated on the lattice.